NES Profile: Physics (308)

Three identical point charges are located on the vertices of an equilateral triangle of side length s.

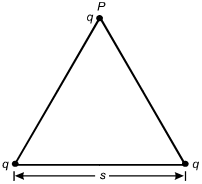
What is the vertical component of the electrostatic force on the charge located on vertex P?

1. ^kq2/_s² sin 30°kq squared over s squared sine 30 degrees
2. ^kq2/_s² cos 30°kq squared over s squared cosine 30 degrees
3. ^2kq2/_s² sin 30°2kq squared over s squared sine 30 degrees
4. ^2kq2/_s² cos 30°2kq squared over s squared cosine 30 degrees

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

D. This question requires the examinee to describe an electric force for a simple charge distribution. From Coulomb's law, the magnitude of the force on q from the left-most charge on the base of the triangle is F = ^kqq/_s²,F equals fraction kqq over s squared, and the component in the vertical direction is ^kqq/_s² cos θ, where θ = 30°kqq over s squared cosine theta, where theta equals 30 degrees. This force points in the positive y-direction. The same applies for the force from the right-most charge. The net force is the superposition of the two forces, which is F = ^2kq2/_s² cos 30°F equals >2kq squared over s squared cosine 30 degrees.

A wire that carries conventional current I in the direction shown is in a magnetic field that points into the plane of the page.

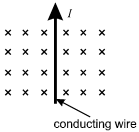
Which arrow shows the direction of the force on the wire?

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

C. This question requires the examinee to analyze the magnetic force on a moving charge in a magnetic field. The diagram shows a magnetic field that points into the page. The wire carries conventional current, which implies positive charges moving toward the top of the page. Since F = Iℓ × B and ℓstart bold F end bold equals I samll l times start bold B end bold and small l represents the direction of the conventional current I, the right-hand rule can be used to cross v into Bstart bold v end bold into start bold B end bold. This shows that the force on the wire points to the left, as shown in response C.

A resistor is connected in series across the terminals of an ideal battery of voltage V. The resistor dissipates power W from the battery. A second, identical resistor is connected in series with the first resistor. What is the total power dissipated by the two resistors?

1. ^W/₂W over 2
2. W
3. 2W
4. 4W

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

A. This question requires the examinee to analyze an electric circuit in terms of energy or power. The power dissipated by the resistor in the circuit is given by P = IV = WP equals IV equals W, where I is the current through the resistor. When a second resistor is added to the circuit, the effective resistance doubles. Since the voltage of the battery is the same, the current is ¹/₂I1 half I. Since there are two identical resistors in the circuit, the voltage across each is ¹/₂V1 half V and the power dissipated by each is P = ¹/₄WP equals 1 fourth W. Therefore, the total power dissipated is P = ¹/₂WP equals 1 half W.