NES Profile: Middle Grades Mathematics (203)

A car is traveling at a speed of 100 kilometers per hour. What is its approximate speed in meters per second?

1. 28 meters per second
2. 36 meters per second
3. 280 meters per second
4. 360 meters per second

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

A. This question requires the examinee to analyze the use of various units and unit conversions within the customary and metric systems. Both units in the given quantity must be converted: kilometers to meters and hours to seconds.

^{100 kilometers}/_{1 hour} • ^{1000 meters}/_{1 kilometer} • ^{1 hour}/_{3600 seconds} = 27.8^meters/_second ≈ 28^meters/_second100 kilometers over 1 hour times 1000 meters over 1 kilometer times 1 hour over 3600 seconds equals 27.8 meters over second which approximately equals 28 meters over second

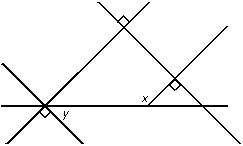
One is horizontal. Four other lines intersect the horizontal line at an unspecified angle that appears to be about 45�. The first line passes through the horizontal line near the left side of the diagram, slanted from upper left to lower right, at point y. The second line passes through point y from lower left to upper right at a right angle to the first line. The third line intersects the second line at a right angle near the top of the diagram. The third line continues toward the lower right, passing through the horizontal line near the right side of the diagram. The fourth line starts at point x on the horizontal line and goes up toward the upper right corner of the diagram, passing through the third line at a right angle.

Five lines intersect as shown. If x and y are angle measures in degrees, which of the following equations relates y to x in the diagram above?

1. y = ¹/₃xy equals 1 third x
2. y = x − 90y equals x minus 90
3. y = ¹/₂xy equals 1 half x
4. y = 180 − xy equals 180 minus x

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

B. This question requires the examinee to analyze properties of points, lines, planes, and angles. If two lines are both perpendicular to the same transversal, the two lines are parallel. Thus there are two pairs of parallel lines in the figure. By the alternate interior angle theorem, x = y + 90°x equals y plus 90 degrees and y = x − 90°y equals x minus 90 degrees.

The end points of one diagonal of a parallelogram are (1, 3) and (1, –3)(1, negative 3). The end points of its other diagonal are (3, 1) and (–1, –1)(negative 1, negative 1). What is the perimeter of the parallelogram?

1. 2 square root of 2 plus 2 square root of 5
2. 10
3. 20
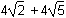4. 4 square root of 2 plus 4 square root of 5

Correct Response and Explanation (Show Correct ResponseHide Correct Response)

D. This question requires the examinee to connect algebra and geometry by applying concepts of distance, midpoint, and slope to classify figures and solve problems in the coordinate plane. Use points (1, 3) and (3, 1), then points (3, 1) and (1, –3),(1, negative 3), and the distance formula to find the length of two different sides of the parallelogram: 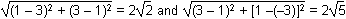the square root of the quantity squared 1 minus 3 plus the quantity squared 3 minus 1 equals 2 square root of 2 and the square root of the quantity squared 3 minus 1 plus left bracket 1 minus left parentheis negative 3 right parenthesis right bracket squared equals 2 square root of 5. There are two of each of these sides, thus the perimeter of the parallelogram is 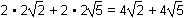2 times 2 square root of 2 plus 2 times 2 squre root of 5 equals 4 square root of 2 plus 4 square root of 5.